Types of study designs
• Meta-analysis
• Systematic review of randomized controlled clinical trials
• Randomized controlled clinical trial
• Prospective controlled cohort study
• Retrospective controlled cohort study
• Prospective uncontrolled cohort study
• Retrospective uncontrolled cohort study
• Case–control observational study
• Case series without a control
• Cross-sectional survey
• Case report
• Anecdotal case report
• Unsupported opinion of expert
The aim of this chapter is to discuss the basic principles of MAs and their conduct and reporting, as well as to investigate the evidence currently existing in the orthodontic literature derived from high-quality studies, such as MAs. The results from these studies will be critically presented and discussed according the specific fields of orthodontics that were investigated. Lastly, the meta-analytic procedures used to obtain conclusions that can be used for practicing evidence-based orthodontics will be evaluated.
6.2 Meta-analysis as a Tool for Evidence-Based Practice
6.2.1 Background
The introduction of MA in medical research has an important positive impact on research synthesis in reopening the question of how best to summarize the results of many separate studies, using statistical procedures for computation of effect size in order to evaluate treatment effects. By combining the data from individual studies, a MA increases the overall sample size to a great extent, which in turn increases the statistical power of the analysis, as well as the precision for estimating the treatment effects. Because the “data” used for MAs are derived from original studies published in scientific journals, the quality of the MAs depends heavily on the quality of these studies. Therefore, issues such as how well these studies were conducted, how well the findings were reported, and how they came to the attention of the meta-analyst are of great importance. Well-performed MAs allow a more objective appraisal of the evidence than traditional narrative reviews, provide a more precise estimate of a treatment effect, and may explain the heterogeneity between the results of individual studies [17]. In contrast, imprecisely conducted MAs may be biased, mainly because of the exclusion of relevant studies or the inclusion of inadequate studies [18]. Clearly, MAs present several advantages over conventional narrative reviews and may constitute a very powerful tool in clinical research. It is, unfortunately, not a perfect tool. Any statistical procedure or analytic approach can be misused or abused. Most of the criticisms of quantitative approaches attempting to reviewing literature are objections to the misuse or abuse of MAs.
6.2.2 Definitions
The term “meta-analysis” was first proposed in 1976 by Glass, a psychologist [15]. He identified three levels of analysis and thereby established a meaningful context for meta-analytic statistical procedures. According to this classificationprimary analysis refers to the original statistical analysis of the data as it is collected by the researchersecondary analysis refers to analysis of this data by someone other than the researcher who collected the original data (possibly for purposes or with analytic strategies other than those of the original researcher), andmeta–analysis refers to analysis of the data of several independent studies.
Meta-analyses are statistical procedures that integrate the results of several independent studies considered to be “combinable” [19]. In other words, a MA provides a logical framework to a research review. This means that similar measures from comparable studies are listed systematically and the available effect-measures are combined, where possible [20]. Trying to define more precisely the term “meta-analysis,” Kassirer [21] stated that “Meta-analyses are studies of studies ….”.
Sometimes MAs are called “statistical overviews” or “systematic reviews”, although the later term should be used only when quantitative data synthesis is not possible. In detail, with a SR a qualitative synthesis of the available data without any statistical analysis is performed when the data derived from the original studies are not similar and they cannot be combined, while with a MA a quantitative data synthesis with specific statistical meta-analytic procedures is attempted when there is data similar enough to be combined. In other words, a MA is a SR with statistical analysis.
6.2.3 Meta-analysis vs. Systematic or Narrative Review Articles
Meta-analyses are similar to SRs because they are based on thorough reviews of the literature about a single research topic. Nevertheless, they differ from these articles, in that theystatistically combine the results of several studies into a single outcome measure. In addition, a significant problem among the narrative review articles is that, although they may start with the same basic aims and may also use the same material in the literature, different conclusions might be reached. Perhaps even more important than the disagreement of conclusions derived from different narrative reviews is the disagreement in conclusions between a narrative review and a MA or a SR of the same research field. For example, Cooper and Rosenthal [22] performed a study with 41 faculty and graduate students who read and integrated the results of the same seven studies of gender differences in task persistence. According to the results of this study, it was found that the traditional reviewers were significantly more likely to conclude that there was no support for the hypothesis tested, in contrast to the generally more accurate meta-analytic reviewers.
6.2.4 Purposes of Meta-analyses
Meta-analyses may have several purposes, such as (a) to summarize a large and complex amount of literature on a topic, thereby resolving conflicting reports, (b) to clarify or quantifying the strengths and weaknesses of various studies on a specific research field, (c) to avoid the time and expense of conducting a clinical trial or in contrary to the need for a major clinical trial, and (d) to increase the statistical power of the analysis by combining data from many smaller studies. By performing such studies, the precision of an estimated treatment effect can be improved, variations in treatment effects through subgroup analysis can be investigated, and the generalizability of known treatment effects can be improved.
6.2.5 Limitations and Strengths of Meta-analyses
Meta-analyses are not without controversies. The benefits and risks of the more complicated procedures of MAs continue to be debated in the medical research community [23–29]. Controversial issues include (a) the ability of the researchers to combine studies that differ in important aspects, such as study populations, experimental designs, and quality controls, (b) the possibility that publication or selection bias could exist when conducting such studies, and (c) the fact that sometimes the results of MAs on the same research topic have been contradictory [20 30]. Meta-analyses present some limitations as well as strengths and advantages. The following paragraphs provide a summary of these aspects [31].
Limitations
Meta-analyses have been accused of oversimplifying the results of a research area by focusing mainly on overall effects while downplaying mediating or interaction effects, and they been criticized that sometimes they are mixing studies that measure “apples” with those that measure “oranges” [32]; ultimately, no meaningful results can be obtained. Some researchers support the idea that MAs ignore the possible impact of study quality and risk of bias on the results of a review. By assessing the quality and risk of bias of the source studies included in the MA, this problem could be eliminated. When conducting a MA, there could be some mistakes in the classification of studies or in calculating the effect sizes because of the complicated coding system used, as well as because many studies do not provide all the necessary information for inclusion in the analyses. A further weakness of MAs can arise from the situations when in some cases the available studies for a particular research area are few in number or they are of low quality, their data are heterogeneous [33], and thus the corresponding results should be interpreted with great caution.
Strengths
The procedures employed in a MA permit quantitative synthesis of the literature that addresses specific research areas. A MA is likely to be more objective than a traditional extensive literature review, and therefore its use is more efficient to summarize large bodies of literature [34]. By means of MA, it is possible to reach stronger conclusions because more studies can be analyzed objectively with specific statistical methods as opposed to traditional narrative literature reviews. Furthermore, it should be mentioned that a MA is also very helpful in examining lack of evidence in a specific research field, providing insight into new directions for research, as well as finding mediating or interactional relationships or trends that they are either too minimal to be observed or they cannot be hypothesized and tested in individual studies [34 35].
6.2.6 Indications and Contraindications of Meta-analyses
When conducting MAs, misleading results could generally be avoided if some basic principles are defined at the outset and carefully followed throughout the investigation. First, the need for performing such a study must be examined, and this need must be later justified in the publication. Victor [36] proposed some indications and contraindications that should be seriously taken into consideration for a MA. According to him, a MA is indicated when: (a) there is a necessity of an urgent decision and because of the lack of time, the performance of a new trial is impossible, (b) there is research on the safety aspects of drugs and other therapies and especially the evaluation of side effects, (c) there are many non-conclusive studies on a specific treatment, where small effects are important and when target-reaching trials are unrealistic in view of needed sample size and time, and (d) there are contradicting results of studies or effects, which vary too much among different types of subjects.
In contrast, MAs are contraindicated when (a) they are performed as the basis of drug approval and registration or (b) they are conducted in an attempt to make an irrelevant or unimportant effect significant by combining numerous insignificant studies showing small effects. In addition, it is also a misuse of MA when a researcher uses it to avoid the time and hard work of conducting a study of his or her own. Some basic principles to overcome these, as well as other, controversial issues are discussed below.
6.2.7 Conducting Meta-analyses
The most important issue before conducting a MA is to define a protocol to work with. This protocol, which will also help to reduce bias, should be precisely followed during the study and should include (a) the definition of the response variables, (b) the methods of literature searching for the individual studies to be included in the analysis, (c) the measures taken to reduce and identify publication bias, (d) the inclusion and exclusion criteria for the studies to be included in the analysis (selection bias), (e) how the data will be extracted from the studies, (f) how the quality (risk of bias) of the source studies will be assessed, and (h) how the data will be analyzed statistically, including among others information concerning the definition of the effect size, the use of fixed effect or random effects model, heterogeneity assessment, as well as subgroup and sensitivity analysis).
Definition of the Response Variables
The definition of the response variables that are intended to be examined is significant, because most of the times different definitions of the same variables (as they are described in the original studies included in the analysis), are not suitable for combining. Furthermore, there is more need to present the minimum difference in the response variable that is considered to be clinically important and not only the statistically significant differences. This way, bias in interpreting the results of the analysis could be avoided, and research is mainly focused on clinical importance.
Methods of Literature Searching
First of all, the period of time covered by the literature search during which the desired studies have taken place must be mentioned, because this places the MA in perspective with developments in medicine that may precede, coincide with, or follow the performed study, and allow other researchers to replicate the study if needed. Nevertheless, the most important issue is a detailed description of the information sources and search strategies that were used to locate the studies to be included in the MA. In MAs, the “material” to be studied consists of individual source studies of identical or similar research fields. It is very important to identify as many of these studies as possible, so that the “sample” used in the MA will be as large and as representative as possible. An incomplete search can lead to “selection bias” by failing to identify important studies. In order to avoid selection bias when conducting a MA, the literature should be searched thoroughly and systematically. It is recommended that these search methods should include: (a) Keyword (index terms) searches of computerized bibliographic databases (such as MEDLINE), which will be described later in this chapter [37]. All additional information about these searches should also be presented, such as the databases searched, the dates covered by the search, and whether or not the search was conducted by a professional medical librarian. (b) Cross-checking citations to appropriate studies, either through reviewing the bibliographies of published articles that have been already identified or through indexing and citation services, such as the Science Citation Index. (c) Checking investigators, granting agencies, and industries or pharmaceutical companies for information on published or unpublished studies. (d) Searching trial registries of pertinent studies, such as The Cochrane Library Databases and the Oxford Database of Perinatal Trials. (e) Journal hand-searching.
In order to identify all possible studies to be included in a MA, not only one but several search strategies should be performed, and computer literature searches should not be the only strategy used to identify studies [20 38 39]. Another question that needs to be answered is if non-English language studies should be included in the analysis [38]. Unfortunately, MAs published in the English language journals restrict their search to papers which were also published in English, although they usually claim a very thorough search to retrieve every paper dealing with the topic to be investigated, which is rather questionable.
Measures to Reduce Publication Bias
The tendency for increased publication rates among studies that show a statistically significant effect of a specific treatment has been already documented [40–42]. Publication bias is correlated to the fact that studies with statistically significant results are more likely to be published than those without statistically significant results [43], and therefore measures should be taken to identify and reduce publication bias.
The inclusion of unpublished data in SRs or MAs is still controversial [20 21 44 45]. However, before conducting a search for a MA, a decision should be made whether unpublished data that have not undergone a formal peer review process, including dissertations or conference presentations, should be included [21 39 42 46].
Finally, publication and selection biases in MAs are more likely to affect small studies, which also tend to be of lower methodological quality. This may lead to “small-study effects,” where the smaller studies in a MA show larger treatment effects. Small-study effects may also arise because of between-trial heterogeneity. Thus, it is generally considered that studies with large sample sizes are less likely to be affected by publication bias than studies with smaller sample sizes, because larger studies are more likely to be reported even when they present negative or not-significant results [38 39].
A very useful graphic method that can be utilized to identify and present possible publication bias in MAs in an association between treatment effect and study size is the “funnel plot” [20 38 39]. This is a scatter plot of the treatment effects calculated from the individual studies on the horizontal axis, against study size or standard error on the vertical axis [47]. Funnel plots take advantage of the well-known “law of large numbers,” i.e., the larger the sample size, the more probable it is that the sample mean is a better estimate of the population mean. This suggests that the precision that results from estimating the underlying treatment effect will increase as the sample size of the component studies increases. Thus, large studies appear at the top of the graph and tend to cluster near the mean effect size. Smaller studies appear towards the bottom of the graph and tend to be dispersed across a range of values.
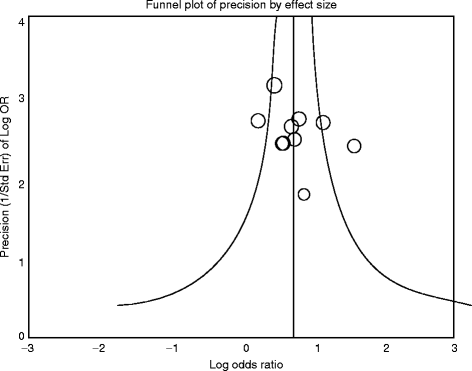

Fig. 6.1
Hypothetical funnel plot. A symmetrical plot indicates the absence of bias (smaller circles indicate smaller studies). Thecircle (study) on the right outside thecurve indicates the possible existence of bias
In the absence of bias the studies are expected to be symmetrically distributed about the pooled effect size (Fig.6.1), and thus visual inspection of a funnel plot may give a clear indication of publication bias. In the presence of bias, the plot’s lower part can be expected to show a higher study concentration on one side of the plot than the other. In case of funnel plot asymmetry, additional statistical tests can be used to further assess the publication bias, such as the Begg & Mazumdar’s rank correlation test [48], the Egger’s test of the intercept [18], the Failsafe N or “file-drawer number” approach [49 50], and the Duval and Tweedie’s “trim and fill” method [51].
Selection Bias
Selection bias is a systematic error that results from the way the subjects are selected into the study or because there are selective losses of subjects before data analysis. Selection bias can occur in any kind of epidemiological study [52] and is one of the main reasons for divergent results among MAs, [39]. Thus, there is significant need to define the specific inclusion and exclusion criteria used for the studies to be included in the MA. Another aspect of selection bias is the populations utilized in the papers to be included in the MA. As MAs combine results of many different studies, the populations involved in these studies may be many and diverse, and there is a need to describe these populations and to which the results are to be generalized. The variability in the populations included in the MA may make interpreting the results difficult.
The inclusion and exclusion criteria of the source studies should be as specific as possible in order to be able to compare only compatible and relevant studies of suitable quality. According to Kassirer [21], the studies included in a MA should meet the following criteria: (a) The included studies should test the same hypothesis [46] and should therefore have the same outcome or end point. (b) These studies should compare similar patients or similar interventions [38 46]. For example, a study testing a drug against a placebo should not be compared with one that tests it against a competing drug. (c) The source studies should present some characteristics of scientific quality, such as adequate sample size, random assignment between treatment and control groups, masking of patients and examiners, quality controls on data collection and management, and finally formal statistical analyses. At least two reviewers should individually perform the selection of studies to be included in the analysis in a blind procedure, and then the inter-reviewer agreement should be objectively evaluated (i.e., by calculating the Cohen’s kappa).
Quality (Risk of Bias) Assessment
Currently the term “risk of bias” assessment is widely used for evaluating each included study in a SR or a MA, while formerly, the authors tended to use the term “quality” analysis. When carrying out a SR or a MA it is important to distinguish between quality and risk of bias and to focus on evaluating and reporting the latter. Assessing the risk of bias should be part of the conduct and reporting of any SR or a MA. In all situations, systematic reviewers are encouraged to think ahead carefully about what risks of bias (methodological and clinical) may have a bearing on the results of their SRs. There are three main ways to assess risk of bias: (a) scales, (b) checklists, (c) and individual component approaches. Scales that are commonly used for the assessment of risk of bias include: the Downs-Blacks scale [53], the Jadad scale [54], and the Newcastle-Ottawa Scale (NOS) as suggested by the Cochrane Handbook for Systematic Reviews of Interventions [55]. Checklists are less frequently used and potentially have the same problems as scales. The most commonly used is the PRISMA checklist [56]. The use of a component approach and one that is based on domains for which there is good empirical evidence and perhaps strong clinical grounds is advocated. The new Cochrane Risk of Bias tool is one such component approach [57].
Characterization of the quality of the studies allows researchers to use more specific inclusion or exclusion criteria and sometimes to assign weights to studies of different quality when combining them in the analysis. At least two reviewers should individually evaluate the quality of each study in a blind procedure, and then the differences among them should be objectively evaluated [38 39] (i.e., by calculating the Cohen’s kappa).
Data Extraction
The criteria used to extract data from various studies included in the MA should be reported and must be specified in advance. In other words, the “who, what, and how” of the extraction process should be precisely described. When the extraction criteria are too general, data extraction could be a subjective procedure, which may introduce bias into the process. Similar to quality assessment of the source studies, data extraction should be performed by two different researchers, and the inter-examiner reliability to establish the consistency of extraction should be evaluated by comparing the results for agreement (i.e., by calculating the Cohen’s kappa).
Statistical Procedures
The effect of statistically pooling the results through a meta-analytic procedure is to increase the sample size. Consequently, the statistical power of the MA is stronger than that of the individual studies. Differences in statistical methods can result in different results, and therefore careful attention should be directed to choosing the statistical procedures for conducting MAs.
There are some basic differences in the statistical procedures used in the source studies and those used in MAs. The most significant difference is the unit of analysis, which in the individual source studies is the subject (e.g., patients, students, and observational entities), while in a MA, it is the results of a study.
Another important difference is the application of appropriate statistical techniques. Some meta-analysts simply apply the usual techniques used for primary analysis also in MAs [58]. As the hypothesis tested in a MA is based on differing sample sizes, these statistical tests will have different sampling variances and will probably violate the assumption of the homogeneity of these variances. Therefore, statistical procedures that are specially developed for MAs seem to be more appropriate for the quantification of the results of the individual studies [59–62].
Despite the existence of these specially designed procedures, some simple statistical methods for combining the results of individual source studies include various counting or summation procedures, such as the “head counting” or “vote counting”approach [32 35 63 64] or simply combining significance tests of the source studies [35]. Researchers should be very careful in choosing these simple procedures for statistical analysis, which are actually not recommended. In the first procedure, the highest number of positive or negative studies determines the results of the analysis. In the second procedure, the results in a MA are summarized by mathematically combining thep-values of each of the studies into a singlep-value. The latter approach is thus based entirely onp-values and because, as mentioned above, studies with nonsignificant results are published less often, this method will be associated with publication bias.
Effect Size
Before conducting a MA, an “effect size”of the variable under investigation should be initially estimated, which combines the differences and standard deviations of the response variables into standardized units across the studies. An effect size is a measure of the strength of the relationship between two variables in a statistical population, or a sample-based estimate of that quantity, and it is calculated from the source data. It is a descriptive statistic that conveys the estimated magnitude of a relationship without making any statement about whether the apparent relationship in the data reflects a true relationship in the population. In that way, effect sizes complement inferential statistics such as p-values. The most commonly encountered effect sizes (a) for continuous data include the Mean difference, the Standardized mean difference, and the Weighted mean difference, (b) for dichotomous data the Odds ratio, the Risk ratio, and the Risk difference, and (c) for censored or survival data the Hazard ratio.
Fixed-Effect and Random-Effects Models
The specific statistical method used depends on several factors, such as the hypothesis being studied and the nature of the response variable. The statistical methods may also involve one of the two types of models, which should be also reported. A “fixed-effect” model makes more assumptions about the variability/heterogeneity in the analysis, while a “random-effects” model makes fewer assumptions about the variability/heterogeneity in the analysis, is more conservative, and thus it may be a better summary of the effects reported in the individual studies of the MA.
Forest plot
A very useful way to report the results of MAs is to present a summary measure of the estimated size and direction of the effect of the treatment with the corresponding confidence intervals by means for example of the odds ratio [65]. This ratio corresponds to the likelihood of an outcome occurring in the treatment group divided by the likelihood that the same outcome will occur in the control group. The procedure can be very clear if it is presented by means of a table and/or a “forest plot of odds ratio” and its95%confidence intervals of each study included in the analysis (Fig.6.2). The studies may be arranged in several ways to reveal certain features of the findings, such as publication date, sample size, and quality of the study. An odds ratio greater than 1 indicates an increased risk in the treatment group, while an odds ratio less than 1 indicates a decreased risk. A ratio of 1 indicates no difference in risk. This means that the outcome is as likely to occur in the treatment group as it is in the control group, and therefore the treatment can be regarded neither as harmful nor as protective.


Fig. 6.2
A “forest plot of odds ratio.” The estimated mean odds ratio and 95% confidence limits are shown for each study. An odds ratio of 1 means that the treatment neither increases nor decreases the risk of the outcome of interest
Heterogeneity or Homogeneity of the Data of the Source Studies
As a MA combines the results of several individual studies, the degree of disagreement or agreement among them (heterogeneity or homogeneity) can also affect the results of the MA. When the source studies present homogeneous data, they can be more easily interpreted; in contrast, heterogeneous data are more difficult to explain. Sometimes, the heterogeneity among the data of the individual source studies is due to the fact that these source studies are conducted without similar protocols [66].
Heterogeneity (and possible causes for it) can be initially assessed by means of visual inspection of the forest plots. However, for a more precise estimate, the Q statistic and the corresponding P-value, as well as the I² index, which is an indicator of true heterogeneity in percentages, should be used. A I² index value of 0% indicates no observed heterogeneity, while larger values show increasing heterogeneity (with 25% indicating “low”, 50% “moderate” and 75% “high” heterogeneity) [58].
Another more useful way to examine and report the degree of heterogeneity between source studies and to identify outliers is theL’Abbé scatter plot (Fig.6.3) [68]. This plot is a representation of event rates in the treated group against those in the control group. If the treatment is beneficial, studies will fall to the right of the line of identity (the no-effect line). A homogenous set of studies will scatter around a parallel line that corresponds to the combined treatment effect.
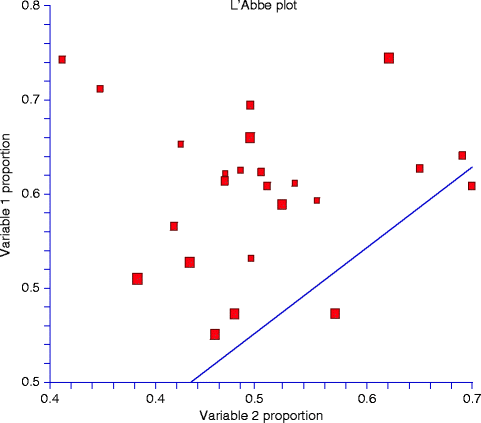

Fig. 6.3
The L’Abbé scatter plot shows the event rates in the treated group (“variable 1 proportion”) against those in the control group (“variable 2 proportion”). If the treatment is beneficial, studies will fall to the right of the line of identity (the no-effect line), which is not the case in the current diagram. In addition, because the set of studies do not scatter around the line, which corresponds to the combined treatment effect, it could be concluded that this set of studies is heterogeneous
Sensitivity Analysis
When important choices and assumptions are made, there is a need to test them with a sensitivity analysis to determine whether their impact on the results is warranted. In a sensitivity analysis, some studies are excluded to determine how their exclusion affects the results. If the effect is great, the studies may have an excessive impact on the results. If the effect is small, the results may be more representative of all the studies.
Software for Meta-analysis
Over the past few years, computer software entirely designed for MAs has increasingly become available, while meta-analytic procedures have been introduced in general statistical software packages. These can be differentiated in commercial or freely available packages and are presented in detail in Tables6.2a and6.2b.
Table 6.2a
Commercial software entirely designed for meta-analysis
|
Software
|
FAST* PRO
|
STATA
|
True Epistat
|
DSTAT
|
Comprehensive meta-analysis
|
MetaWin
|
|---|---|---|---|---|---|---|
|
Operating system
|
MS-DOS
|
Windows
|
MS-DOS
|
MS-DOS
|
Windows
|
Windows
|
|
Distributor
|
Academic Press, 24–28 Oval Road, London NW1 7DX UK
|
Timberlake Consultants, 47 Hartfield Crescent, West Wickham, Kent BR4 9DW UK
|
Epistat Services, 2011 Cap Rock Circle, Richardson TX 75080–3417 USA
|
Lawrence Erlbaum Associates, 365 Broadway, Hillsdale NJ 07642 USA
|
Biostat, 14 North Dean Street, Englewood, NJ 07631 USA
|
Sinauer Associates, 23 Plumtree Road, Sunderland, MA 01375–0407 USA
|
|
Internet address
|
Table 6.2b
Freely available software entirely designed for meta-analysis
|
Software
|
RevMan
|
Easy MA
|
Meta-analyst
|
|---|---|---|---|
|
Operating system
|
Windows
|
MS-DOS
|
MS-DOS
|
|
Distributor
|
The Cochrane Collaboration
|
Dr. Michel Cucherat, Department of Clinical Pharmacology, 162 Avenue Lacassagne, F-69003 Lyon, France
|
Dr. Joseph Lau, New England Medical Center, Box 63, 750 Washington Street, Boston, MA 02111 USA
|
|
Internet address
|
http://ims.cochrane.org/revman
|
Available by e-mail: joseph.lau@es.nemc.org
|
6.2.8 General Suggestions
In summary, when conducting a MA, there are some general rules that should be followed. Investigators are advised to:
-
Do everything for the right cause and be prepared to defend it.
-
Perform the procedures of statistical analysis in more than one way, whenever it is possible.
-
Report everything (also in the form of tables or diagrams) in order to provide readers, reviewers, or editors with all the information they would need to evaluate the meta-analytic procedures followed.
-
Finally, do everything to minimize mistakes and/or biases.
6.3 Meta-analysis in Orthodontic Literature
The first MA assessing the effect of a therapeutic intervention was published in 1955 and evaluated a placebo treatment [69]. Subsequently, the development of more sophisticated statistical techniques took place in the social sciences [70–73]. Meta-analysis was rediscovered by medical researchers for use mainly of the RCT research, particularly in the fields of cardiovascular disease [74–76], oncology [77 78], and perinatal care [79]. Furthermore, MAs of observational studies [80] and “cross-design synthesis” [81 82] have also been performed.
The number of papers published utilizing MAs in medical research has increased sharply during the last 10 years, and in the year 2003 represented 0.23 % of all published studies (Fig.6.4 and Table6.3). Despite the large number of MAs in medical research only 18 such papers were published (as cited in MEDLINE) in the field of orthodontics until the year 2003 (Tables6.3 and6.4). However, it must be emphasized that MAs are difficult to conduct in orthodontics because of the nature of the available literature [83].
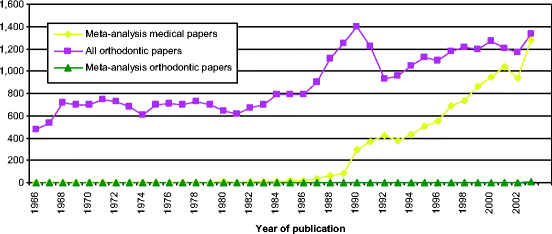

Fig. 6.4
Diagram presenting the number of meta-analyses indexed in MEDLINE during the period 1966–2002 (Results from MEDLINE search using title word or medical subject heading [publication type] “meta-analysis”)
Table 6.3
Number of meta-analyses as indexed in the MEDLINE database during the period 1966–2002
|
Year
|
All papers indexed
|
Publications about meta-analysisa (%)
|
Orthodontic publicationsb
|
Orthodontic publications about meta-analysisa,b
|
|
|---|---|---|---|---|---|
|
2003
|
568,232
|
1,279
|
0.23
|
1,336
|
5
|
|
2002
|
506,395
|
937
|
0.19
|
1,166
|
2
|
|
2001
|
516,499
|
1,039
|
0.20
|
1,207
|
2
|
|
2000
|
510,653
|
951
|
0.19
|
1,268
|
0
|
|
1999
|
471,569
|
865
|
0.18
|
1,199
|
2
|
|
1998
|
455,432
|
732
|
0.16
|
1,215
|
2
|
|
1997
|
437,230
|
690
|
0.16
|
1,175
|
1
|
|
1996
|
440,734
|
551
|
0.13
|
1,090
|
1
|
|
1995
|
430,932
|
509
|
0.12
|
1,119
|
0
|
|
1994
|
420,457
|
433
|
0.10
|
1,049
|
1
|
|
1993
|
410,592
|
374
|
0.09
|
955
|
0
|
|
1992
|
402,381
|
424
|
0.11
|
932
|
1
|
|
1991
|
399,002
|
368
|
0.09
|
1,220
|
1
|
|
1990
|
397,165
|
293
|
0.07
|
1,396
|
0
|
|
1989
|
390,099
|
86
|
0.02
|
1,247
|
0
|
|
1988
|
374,047
|
63
|
0.02
|
1,109
|
0
|
|
1987
|
355,824
|
34
|
|||
Stay updated, free dental videos. Join our Telegram channel

VIDEdental - Online dental courses


