Introduction
Deflections of the lever arms on 3 commercially available miniplates (Stryker, Kalamazoo, Mich; KLS Martin, Tuttlingen, Germany; and Synthes, West Chester, Pa) were compared to determine whether orthopedic forces produce permanent deformation.
Methods
Thirty-six miniplates were tested on a load frame in this ex-vivo trial. The force level at which permanent deformation occurred was measured. The arms of the miniplates were extended beyond the force level needed for orthopedic anchorage.
Results
Load-displacement data followed an expected trend from beam theory, where a linear region of elastic response was followed by an elastic-plastic transition to fully plastic deformation. The yield was measured at the beginning of the nonlinear response. Beam bending analysis was used to determine the yield stress of each sample and to estimate the elastic modulus. In some cases, the calculated yield stresses were higher and the modulus of elasticity was lower than the reported values for titanium. This was due to the torsion in the testing. Loads at the point of initial yielding ranged from 1280 to 3000 g, depending on the miniplate type. Stryker’s yield was statistically lower than that for KLS Martin and Synthes, which were well above the ranges needed for orthopedic forces.
Conclusions
All 3 miniplates were capable of withstanding orthopedic forces. The 3 brands had significant differences in modulus of elasticity values, which were lower than published values.
Orthodontists treating skeletal discrepancies in preadolescents can take advantage of growth to modify jaw size or position inconsistencies; it is widely believed that the application of additional force to the sutures allows for separation and osseous remodeling. This, in theory, can be applied to any jaw discrepancy to modify excessive Class II, Class III, or facial height differences.
Hickory and Nanda applied wire springs to the sutures of rats. They found that low forces (0.2-0.6 g) could generate cellular activity along with increased sutural width. Therefore, it is widely believed that applying forces can cause growth modification. The question is what range of force is optimal for growth modification?
Although optimal forces for overall bone reconstruction are not known with certainty, an example of an optimal force for orthopedic movement can be evaluated in regard to protraction of the maxilla. It is generally believed that, as a child ages, the sutures become more intertwined and more tortuous, therefore making it more difficult to open the suture. This suggests that higher forces are necessary as a child ages. It is also generally believed that the applied orthopedic force should be higher than the dental force; currently, optimal dental forces can vary.
Therefore, most information on the actual force magnitude for orthopedic movement is based on clinical experience or is anecdotal. Krishnan and Davidovitch suggested greater than 300 g. Nanda advised using forces for protraction of the maxilla between 200 and 800 g; greater than 1500 g has been suggested in other studies.
To apply this force to the maxilla, generally, protraction facemask therapy or reverse-pull headgear is used. These original maxillary protraction appliances involved forces applied to the dentition for transmission to the sutures. Usually, these appliances are attached to the molars, to take advantage of their larger roots for better anchorage. Additionally, sometimes maxillary teeth are splinted together as a single unit with or without a rapid palatal expander or a bonded rapid palatal expander to provide additional anchorage. There have been conflicting reports as to whether a rapid palatal expander is needed. Splinted or nonsplinted, the use of teeth for anchorage can result in unwanted consequences to the dentition and facial appearance, such as extrusion of the teeth, downward rotation of the mandible, and increased vertical dimension of the face.
Since tooth movement can detract from orthopedic movement that might occur during treatment, it is advantageous to apply forces directly to the bone, rather than to the dentition. Deliberately, ankylosed deciduous canines were used by Shapiro and Kokich to provide anchorage for maxillary protraction. They found 3 mm of change in 1 year with minimal dentoalveolar movement.
Skeletal anchorage systems were simultaneously developed to provide anchorage similar to that of ankylosed deciduous canines; they began by evolving from osseointegrated dental implants. They soon matured into surgical mini-implant systems made specifically for orthodontics. These included titanium plates secured by miniscrews that had been used for reconstructive surgery. Soon after, there were successful trials by Umemori et al in which molar intrusion was facilitated by recontouring the surgical plate with a lever arm that extended into the oral cavity. Later, in a prospective study, Cheng et al demonstrated that miniplates could be used to provide stable anchorage in orthodontic tooth movement.
Smalley et al applied orthopedic forces (approximately 600 g) on osseointegrated implants to protract the maxilla with success in monkeys in 1998. This was the first trial that involved the use of implants for orthopedic movement in animals. Singer et al in a case report demonstrated that protraction of the maxilla was possible using osseointegrated implants attached directly to the zygomatic processes of the maxilla.
After the experiment of Singer et al was published, Enacar et al used a lag screw in the maxillary alveolus, and Hong et al used a midpalatal onplant for protraction. Afterward that, Kircelli and Petkas, and Kircelli et al integrated previous miniplate techniques directly to the protraction facemask and applied these for orthopedic reconstruction without the dentition. DeClerck et al were able to achieve significant growth modification using miniplates placed bilaterally between the mandibular canines and first premolars and in the maxillary infrazygomatic crests. They then engaged Class III elastics to allow for growth modification without the dentoalveolar repercussions normally seen with a protraction facemask.
Additionally, other implant-supported skeletal movement techniques have since been developed. For example, a jackscrew attached to skeletal anchors, also known as a bone-borne expander, allows for maxillary expansion even when the teeth are delayed or missing.
Once again, these bone-borne expanders bring into question what amount of force is optimal for sutural expansion. As a child ages, more force must be applied to the sutures to allow expansion across the suture. If greater forces can be applied directly to the suture without causing dentoalveolar consequences, sutural expansion might be attempted more routinely in the future in nearly ossified sutures such as those in adolescents and adults. This would require greater forces applied directly to the skeletal anchorage system and questions whether miniplates can withstand such forces. Although there are no known failures because of miniplates succumbing under increased forces, a prospective study should be conducted before failure occurs in a clinical setting with patients. In this study, we sought to answer whether miniplates can withstand current orthopedic forces without permanent deformation of the lever arm.
Material and methods
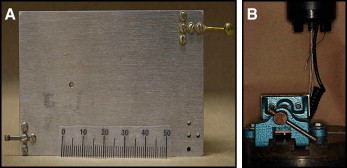
We sought to determine the range of forces that can be applied to a miniplate before permanent deformation of the lever arm occurs. Miniplates (all FDA approved) from 3 manufacturers were tested: Synthes (Kalamazoo, Mich), KLS Martin (Tuttlingen, Germany), and Stryker West Chester, Pa), as shown in Figure 1 . The miniplates were screwed and mounted on a stainless steel block and placed in a lock vise to perform our tests ( Fig 2 , A ). Each plate was horizontally leveled in the upper right corner of the vise 2 mm from the top and side for the test. The forces were applied in a vertical direction and read on a testing machine (model 5566, Instron, Canton, Mass) as shown in Figure 2 , B . Force was applied to the miniplates continuously from 0 to 100 N at a rate of 1 mm per minute as done by Carano et al in a study on miniscrews. A total of 36 miniplates were tested, 12 from each group. The corresponding part numbers and material properties are listed in Table I .

| KLS Martin | Synthes | Stryker | |
|---|---|---|---|
| Product description | C-tube plate with 6–mm bridge | OBA domed design, 4 holes | Leibinger T-shaped plate with 5 holes |
| Part number | 25-301-03 | GP2602-A | 5505163 |
| Lot number | 30482338 | 5889912 | E18255051639E |
| Total length of body (mm) | 6.4 | 8.3 | 13.1 |
| Dimensions of body in first section (mm) | 3.2 × 3.2 | 13.5 × 4.5 | 11.7 × 3.8 |
| Dimensions of body in second section (mm) | 11.1 × 3.2 | 4.5 × 4.5 | 9.3 × 3.8 |
| Thickness of body (mm) | 0.66 | 0.76 | 0.65 |
| Shape of arm | Rectangle | Rectangle | Two tubes fused together with the thicker tube closer to the body |
| Total length of arm (mm) | 5.3 | 11.8 | 9.01 |
| Total width of arm (mm) | 1.59 | 1.71 | 1.08 × 1.08 × 3.8 for first section |
| Total thickness of arm (mm) | 0.66 | 0.76 | 0.86 × 0.86 × 5.2 for second section |
| Size of head (mm) | 2.6 × 2.3 × 2.5 | 4.47 × 4.47 × 2.5 | 4.00 × 4.00 × 4.45 |
| Material | F67 grade 2 titanium | Commercially pure grade 4 titanium | Combination of commercially pure titanium and titanium alloy |
There are different grades of titanium; the most common are 1 through 4, which are considered commercially pure and unalloyed. The differences in grade are caused by the amount of interstitial elements in the titanium. Generally, the tensile and yield strengths go up with the grade number for these “pure” grades. KLS Martin samples are made from grade 2 titanium, and Synthes samples are made from grade 4 titanium.
KLS Martin samples are composed of grade 2 F67, which implies that they follow the American Society for Testing and Materials standard specifications for unalloyed titanium in surgical implant applications. It is unclear whether Synthes and Stryker use the same American Society for Testing and Materials standard.
Three mechanical property values are critical for defining the clinical usefulness of miniplates: modulus of elasticity, and the load and maximum normal stress at the proportional limit. The slope of the force-deflection curve is proportional to the modulus of elasticity or stiffness. The elastic modulus is calculated by using bending mechanics of the miniplates during the applied tensile loading at the end of the beam. At the proportional limit, the miniplate reaches a transition to elastic-plastic bending and is observed at the initiation of a nonlinear load-displacement response. Loads are recorded at the proportional limit and indicate the initiation of plastic (permanent) deformation in the material. The maximum normal stress on the surface of the miniplate arm cross-section is calculated from the load at the proportional limit and represents the yielding stress of the material. Results were observed and calculated for each plate that was tested. No plates were tested to the failure point or ultimate tensile strength because of the constrictions of the testing machine’s load cell. However, after large-scale plastic deformation, distinct regions were present during the test where an increase in stress was observed once the wire was fully bent. At this point, the instrument was measuring the compliance of the miniplate body attached to the rigid support.
Results
Measurements were made on 12 samples each from 3 manufacturers: KLS Martin, Stryker, and Synthes. The sample means of the yield load, the maximum stress at the proportional limit, and the elastic modulus for each brand were compared. The results are shown in Table II .
| Synthes | KLS Martin | Stryker | |
|---|---|---|---|
| Modulus of elasticity (GPa) | |||
| Mean (SD) | 43.5 (6.08) | 23.12 (4.01) | 106.52 (35.23) |
| Median | 44.41 | 22.75 | 103.57 |
| P value | <0.0001 | ||
| Yield load (N) | |||
| Mean (SD) | 29.01 (1.97) | 27.63 (3.30) | 12.89 (3.44) |
| Median | 29.5 | 26.66 | 12.61 |
| P value | <0.0001 | ||
| Maximum normal stress (MPa) | |||
| Mean (SD) | 929.56 (63.00) | 783.70 (94.20) | 1015.1 (271.2) |
| Median | 945.08 | 756.31 | 993.12 |
| P value | 0.0046 | ||
Stay updated, free dental videos. Join our Telegram channel

VIDEdental - Online dental courses


